Skip to content
Technical University of Munich

Quantum Computing and Pattern Recognition

 Recently, we realized a novel quantum pattern recognition scheme, which combines the idea of a classic Hopfield neural network with quantum adiabatic computation. In contrast to classic neural networks, the algorithm can simultaneously return multiple recognized patterns. The approach also promises extension of classic memory capacity. A proof of principle for the algorithm for two qubits is provided using a liquid state NMR quantum computer.

Together with researchers from Siemens Corporate Technology (CT), we have achieved the first experimental implementation of an artificial neural network on a simple quantum computer. The simulation of pattern recognition conducted by the CT researchers was evaluated with a nuclear magnetic resonance spectrometer (NMR) at the TU München.

The patterns used are composed of pixels, and each pixel can assume two colors, which are depicted by means of qubits. Using special algorithm, simulations can predict how a real quantum processor would behave if confronted with a new color pattern. The algorithm compares the patterns with patterns stored in the memory and indicates the degree of similarity.

For the experiments at the TU München, a solution of sodium formiate at room temperature was used. Each sodium formiate molecule contains one carbon and one hydrogen atom. In a strong magnetic field, each of the two particles forms one quantum bit (qubit). The measured signals of the actual quantum computer corresponded exactly to the signals that had previously been predicted by numerical simulations.

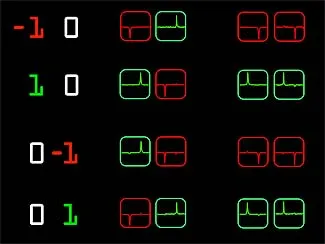
The simple stored patterns consisted of the values 1 (green) and -1 (red). The left colum shows incomplete input patterns. The experimentally recognized complete patterns (and their spectral signatures) are shown in the middle column for the case, where the stored patterns are (1,-1) and (-1,1). The right colums show the results for the case, where the stored patterns are (1,1) und (-1,-1).

Photo: Siemens

Rodion Neigovzen and Prof. Dr. Steffen Glaser in the NMR-laboratory at TU München.

Original manuscript:

quant-ph: Quantum Pattern Recognition With Liquid State NMR by R. Neigovzen, J. Neves, R.Sollacher, S. J. Glaser

Further information:

 

Further publications in the field of quantum information processing by the Glaser group:

  • Optimal Control for Generating Quantum Gates in Open Dissipative Systems
    T. Schulte-Herbrüggen, A. Spörl, N. Khaneja, S. J. Glaser
    preprint: quant-ph/0609037.
  • The Significance of the C-Numerical Range and the Local C-Numerical Range in Quantum Control and Quantum Information
    T. Schulte-Herbrüggen, G. Dirr, U. Helmke, S. J. Glaser
    Lin. and Multilin. Alg. 56, 3-26 (2008).
  • Geodesics for Efficient Creation and Propagation of Order along Ising Spin Chains
    H. Yuang, S. J. Glaser, N. Khaneja
    Phys. Rev. A 76, 012316/1-6 (2007). Preprint (pdf)
  • Shortest Paths for Efficient Control of Indirectly Coupled Qubits
    N. Khaneja, B. Heitmann, A. Spörl, H. Yuan, T. Schulte-Herbrüggen, S. J. Glaser
    Phys. Rev. A 75, 012322/1-10 (2007). Preprint (pdf)
  • Optimal Control of Josephson Qubits
    A. Spörl , T. Schulte-Herbrüggen, S. J. Glaser, V. Bergholm, M. J. Storcz, J. Ferber, F. K. Wilhelm
    Phys. Rev. A 75, 012302/1-9 (2007).
  • Parallelising Matrix Operations on Clusters for an Optimal Control-Based Quantum Compiler
    T. Gradl, A.K. Spörl, T. Huckle, S. J. Glaser, and T. Schulte-Herbrüggen
    Proc. EUROPAR 2006, Parallel Processing Lecture Notes in Computer Science 4128, 751-762 (2006).
  • Non-Computing Applications of Quantum Information in NMR
    S. J. Glaser, T. Schulte-Herbrüggen, N. Khaneja
    Informatik Forsch. Entw. 21, 65-71 (2006).
  • Quantum Computing Implemented via Optimal Control: Theory and Application to Spin- and Pseudo-Spin Systems
    T. Schulte-Herbrüggen, A. K. Spörl , R. Marx, N. Khaneja, J. M. Myers, A. F. Fahmy, S. J. Glaser
    in Lectures on Quantum Information, Eds.: D. Bruß and G. Leuchs, pp. 481-501, Wiley-VCH (2006).
  • From Network Complexity to Time Complexity via Optimal Control
    T. Schulte-Herbrüggen, A. Spörl, N. Khaneja, S. J. Glaser
    NATO Science Series,III: Computer and System Sciences 199, 353-357 (2006).
  • Relaxation Optimized Transfer of Spin Order in Ising Chains
    D. Stefanatos, N. Khaneja, S. J. Glaser
    Phys. Rev. A 72, 062320/1-6 (2005). Preprint (pdf)
  • Optimal Control-Based Efficient Synthesis of Building Blocks of Quantum Algorithms Seen in Perspective from Network Complexity towards Time Complexity
    T. Schulte-Herbrüggen, A. Spörl, N. Khaneja, S. J. Glaser
    Phys. Rev. A 72, 042331/1-7 (2005). Preprint (pdf)
  • Increasing the Size of NMR Quantum Computers
    S. J. Glaser, R. Marx, T. Reiss, T. Schulte-Herbrüggen, N. Khaneja, J. M. Myers, A. F. Fahmy
    in: Quantum Information Processing, pp. 53-65, Eds.: G. Leuchs, T. Beth (Wiley-VCH).
  • Broadband Geodesic Pulses for Three Spin Systems: Time-Optimal Realization of Effective Trilinear Coupling Terms and Indirect SWAP Gates (81)
    T. O. Reiss, N. Khaneja, S. J. Glaser
    J. Magn. Reson. 165, 95-101 (2003). Preprint (pdf)
  • Sub-Riemannian Geometry and Time Optimal Control of Three Spin Systems: Quantum Gates and Coherence Transfer
    N. Khaneja, S. J. Glaser, R.
    Brockett Phys. Rev. A 65, 032301 (2002).
  • Efficient Transfer of Coherence Through Ising Spin Chains
    N. Khaneja, S. J. Glaser
    Phys. Rev. A 66, 060301(R) (2002).
  • Geometry of Quantum Computing by Hamiltonian Dynamics of Spin Ensembles
    T. Schulte-Herbrüggen, K. Hüper, U. Helmke, S. J. Glaser
    in: Applications of Geometric Algebra in Computer Science and Engineering, L. Dorst, C. Doran and J. Lasenby, Eds., Birkhäuser, Boston, 269-281 (2002).
  • Cartan Decomposition of SU(2n), Constructive Controllability of Spin Systems and Universal Quantum Computing
    N. Khaneja, S. Glaser
    Chem. Phys. 267, 11-23 (2001).
  • Time Optimal Control in Spin Systems
    N. Khaneja, R. Brockett, S. J. Glaser
    Phys. Rev. A 63, 032308 (2001).
  • NMR Quantum Computing
    S. J. Glaser
    Angew. Chem. Int. Ed. 40, 147-149 (2001); Angew. Chem. 113, 151-153 (2001).
  • Rapid Solution of Problems by Nuclear Magnetic Resonance Quantum Computing
    J. M. Myers, A. F. Fahmy, S. J. Glaser, R. Marx
    Phys. Rev. A 63, 032302 (2001).
  • Chemical Enigneering of an NMR Quantum Computer
    R. Marx, A. F. Fahmy, J. M. Myers, W. Bermel, S. J. Glaser
    in: Quantum Computing, Eds.: E. Donkor and A. R. Pirich, Proceedings of SPIE Vol. 4047, pp. 131-138 (2000).
  • Nuclear Magnetic Resonance Quantum Computing Exploiting the Pure Spin State of Para Hydrogen
    P. Hübler, J. Bargon, S. J. Glaser
    J. Chem. Phys. 113, 2056-2059 (2000).
  • Approaching Five-Bit NMR Quantum Computing
    R. Marx, A. F. Fahmy, J. M. Myers, W. Bermel, S. J. Glaser
    Phys. Rev. A 62, 012310 (2000).
  • quant-ph/9905087 press reports Unitary Control in Quantum Ensembles, Maximizing Signal Intensity in Coherent Spectroscopy
    S. J. Glaser, T. Schulte-Herbrüggen, M. Sieveking, O. Schedletzky, N. C. Nielsen, O. W. Sørensen, C. Griesinger
    Science 280, 421-424 (1998). (Abstract and additional information)
Photo by Volker Steger/ Science Photo Library

Nuclear magnetic resonance (NMR) spectrometer at the TU München

Molecular "hard ware" of the first five-qubit NMR quantum computer Preprint: quant-ph/9905087 press reports

To top